The Setup
As a first example, I'll discuss a particular pet-peeve of mine, which is something covered in many introductory quantum mechanics classes: The algebraic solution to quantum (1D) simple harmonic oscillator.1 The one-dimensional, time-independent Schrödinger equation is:
(1)
where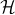 
is the Hamiltonian of the system. Explicitly, this Hamiltonian is
(2)
where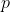 
is the particle's momentum, 
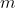is it's mass and 
is the potential the particle is placed into. The potential associated with a classical harmonic oscillator is
(3)
where 
. For the sake of convenience, so we don't get bogged down with various factors,2 we'll consider 
. Then, if we substitute (3) back into (2) we write the Hamiltonian as:
(4)
A bad way
Figure 1: How Einstein developed his famous 
expression.
Now, at this point, many texts, (see [1] or [2] for example,) define, with no motivation other than future ``convenience'', two operators
(5a)
(5b)
and proceed to show how these can be used to simplify the Hamiltonian and easily solve the problem. While it is an elegant and quick solution, this presentation is completely useless. I find it highly unlikely that Dirac sat down to solve this problem and tried a whole series of random operators
and so on, along with their complex conjugates, until he lucked out with the solution in (5), see Fig. 1.
A better way
What is far more likely is the argument given by Griffiths in [3], which I'll loosely follow. He presents a rationale and a method for approaching this problem. Namely, he suggests factoring the Hamiltonian (4) into terms linear in 
and 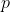. If we ignore the operator properties of and 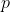momentarily, and consider the classical quantities, we can factor the Hamiltonian
(6)
Now we see a reason why (5) makes sense to try. Each term, either on the right or left side of (6),3 contains two terms which are complex conjugates of each other. If this were a classical problem, we could, in principle, make a change of variables converting the Hamiltonian to something of the form
(7)
where 
is any of the combinations of and 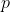in (6). Although this is not a ``canonical transformation,'' the symmetric form4 of the Hamiltonian allows us to reduce the Hamiltonian from a function of two dynamical variables to a function of a single dynamical variable.
Switching back to quantum mechanics, we now see a rationale for choosing
References
[1] J.J. Sakurai. Modern Quantum Mechanics. Addison-Wesley, San Francisco, CA, revised edition, 1993.
[2] F. Schwabl. Quantum Mechanics. Springer, 3rd edition, 2005.
[3] D.J. Griffiths. Introduction to Electrodynamics. Pearson Prentice Hall, 3rd edition, 1999.
[4] P.A.M. Dirac. The Principles of Quantum Mechanics. Oxford University Press, USA.
1 I'll be discussing this in the boring algebraic sense of symbols and whatnot, leaving off the geometric/visual interpretation of the algebra for another time.
2 Or maybe I'm just lazy
3 And, since this is a classical problem, the order does not matter, either.
4 Yes, it's only up to a constant which I've set to one, but you can still symmetrize things by changing to unitless variables, see [2].
5 Recall that the
6 It actually does not matter to the solution of the problem. The only change is which operator acts to `step up' the state. Dirac actually defined his operators with the
